Click here for the Standard Celeration Chart.J
Precision teachers and standard celeration charters will quickly point out that kindergartners (Bates & Bates, 1971) senior citizens (Kubina, Haertel, & Cooper, 1994) and everyone in between can successfully understand and use the standard celeration chart.
This guide does not focus on explaining why persons should use precision teaching or standard celeration charting, Rather, this guide will show interested readers how to chart and by doing so, Many readers will grasp precision teaching standard celeration charting without reading books or articles by virtue of measuring charting behavior. For those, however interested in reading more about precision teaching and standard celeration charting please consult the following sources: (Binder, 1996; Lindsley, 1992, 1993; Maloney, 1998; McGreevy, 1983; Pennypacker, Koenig, & Lindsley, 1972; Potts, Eshleman, & Cooper, 1993; White, 1986; White & Haring, 1980).
WHAT DO ALL THOSE LINES MEAN?
To begin to understand the chart one must first figure out what the horizontal and vertical lines mean. I will cover some figures on the standard celeration chart (SCC) that will help you understand what the lines mean. Then I will cover what we put on the chart.
VERTICAL LINES (DAY LINES)
We call the vertical lines “day lines.” Why? Each one represents a day (I bet you saw that one coming!) At the bottom of the chart you see the following figure:
![]()
This figure helps you quickly orient to the days. You see 0, 14, 28 and so on up until 140. The 140 means 140 days appear on the chart. (Factoid: The 140 days, which comes out to 20 weeks, came about to accommodate a public school semester). Now that you know the function of day lines, to actually make the days meaningful for you or a learner we must assign the day lines a staring point. Note on the top of the chart you see the following

For personal use the easiest way to set up the times for chart follows: the Sunday (the actual date) before you started charting goes in the first Day/MO/YR category. For instance, let’s say I started to chart my keyboarding skills on January 20 in 2000. It so happens that January 20 in the year 2000 falls on a Thursday. So to find that Thursday I must set up my chart by writing in the preceding Sunday (I do this because the chart Starts at Sunday and has 140 days that follow it). Now I must find the date of the Sunday before January 20. That date, January 16, will now go in the first Day/MO/YR category. We use the following convention to for the Day/MO/YR category. We represent Day by a number (in our case a 16 for the 16th of January). For MO, or month we use the 3-letter abbreviation (we would then represent January as Jan). Last we show the year by a two symbol number (we would show 2000 as 00). So I would write this in the first Day/MO/YR category to show the preceding Sunday to our charting:
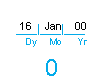
Now we can easily find the first day we charted, Thursday January 20. The figure below shows how we move over from the first day, Sunday January 16, 2000 to Thursday January 20, 2000 (the arrow points to Thursday, the 20 of January 2000).

When I made the preceding figure I made the dark, Sunday lines thick so you can readily distinguish them from the other days. If you look on a typical daily Standard Celeration Chart you will see the Sunday lines do appear thicker than the other lines (not as thick I made them!). This will help you quickly get your bearing on the chart.
Time for a quiz (you will need a calendar for this)! We just set up our chart to show the first as 16 January 2000. Question 1: What do we write in the next Day/MO/YR? Question 2: What does the 4 under the Day/MO/YR category mean?

I like working with you because of quickly you pick these things up! Yes, I bet you answered both questions correctly. The first answer, we must write in the date for the next 28 days after 16 Jan 00. We write the date February 6, 2000 the same way we write the date for all other Day/MO/YR (two digit day, 3 letter month abbreviation, and two digit year). The following figure shows how to write in the Day/MO/YR category following the first Day/MO/YR:

As you correctly deduced the second question, that 4 stands for 4 weeks (or 28 days). So I suppose if I asked you what the 8, 12, 16, and 20 stood for you could easily reply “the number of weeks passed by since the first week.” I knew you could get this stuff! Good job! Now you can find any day on the chart just by figuring out where that day lies in relation to the first Sunday before we began charting
Technical note: Looking at the bottom of the chart I supplied you will recall the bottom figure goes 0, 14, 28, 42, 56, 70, 84, 98, 112, 126, 140. That number tells you how many days have passed from the first Sunday. Therefore 28 means 28 days have passed and 70 means 70 days have passed. If you have tried to follow along this guide with an old Standard Celeration Chart you will notice a discrepancy here. The older version, called DC-9EN, will have numbers at the bottom of the chart as follows 0, 10, 20, 30, and so on up to 140. These numbers do match up with the Day/MO/YR numbers (the charts I sent you) at the top in the newest version of the SCC (Dpmin-11EC): 0, 14, 28 etc. up to 140.
Now that you understand vertical lines we will move onto the horizontal line or frequency lines. We use the term frequency lines because the horizontal lines display frequency. Oh wait, I neglected to explain frequency! (Often times in my excitement I sometimes forget to explain the details so please bear with me).
Frequency represents a unit of measurement. You probably know of many frequencies. For example, when you drive your car the speed at which you travel depicts a frequency: miles per hour. If you traveled 60 miles per hour (or kilometers per hour for our good friends beyond the States) you find 2 elements that make up a frequency. (1.) Measurements of a physical event, in this case the distance of 60 miles and (2) a time frame. Our time frame encompasses one hour. So the frequency looks like this:
60 miles
hour
If you want to figure out the frequency of any behavior or skill you can use the following formula:
Number of events
Time frame
Let’s say I want to know how many words I can orally (see the text and say the words – see/say) read in one-minute. I could easily figure out my see/say reading frequency by doing the following steps. I get a countdown timer (that means a timer you can set for one-minute which will count down to zero- also it helps that the countdown timer beeps when it reaches zero) and set it for one-minute. Then I get a book. I start the timer and then start to see/say words. Once the count down timer beeps I count up how many words I read correctly in the one-minute timing period. Viola! I now have a frequency! I read 223 words correctly in one-minute.
Taking frequency measurements set precision teaching apart from most other educational measurement practices. The most common way to measure how well someone does something typically occurs with percentage. You remember percentage, 90 to 100% equals a an “A,” 80 to 89% equals a “B” and so on. One problem with using percentage readily appears when you try to distinguish competence. For instance I just shared with you the frequency of my see/say reading performance. Let’s pretend I gave the same passage I read to another person who could see/say (or orally read) 110 words in one-minute. If we converted my frequency, and my imaginary friend’s frequency, to percentage correct we both would get 100%! Wait a minute! My imaginary friend read less than half of what I did in one-minute therefore our competency must differ! Percentage masks how well people perform tasks and report proficiency on a very broad level. The good news; if you use percentage you can quickly figure out a frequency simply by recording the time it takes a person to do something in. So if we did a spelling test and previously reported the results in percentage correct (e.g., 90%, 76% etc.) we need to record the time it took the person to spell the words. Then we have a frequency. For instance a young man got a 90% (9 correct out of 10 words) on his spelling test. When we record the time it took to spell the words we now have a frequency: 9 words spelled correctly in 5 minutes (we also have a frequency for incorrectly spelled words, 1 words spelled incorrectly for 5 minutes). The frequency measure gives us an enormous amount of information compared to percentage (moral of the story: use frequency whenever you can).
Continued on Page 2